This view on contingent episodes prompted us to consider how we could engineer both the variety and the difficulty of the problem-situations, to provide more opportunities for the teacher to learn whilst teaching. Thus we sought to design a classroom setting (or at least the potential for one) in which teachers could effectively tackle the not too difficult problem-situations in the moment of instruction. In addition we wanted to move the too difficult problems out of the classroom and into the planning phase, before the lesson. To accomplish this aim we attempted to restrict the variety, and reduce the demands of the problem-situations. This we did by developing a range of pre-designed ‘student’ responses to a variety of mathematical problems. Figure 1 is one example. The resources are designed so that students first attempt to solve the problem by themselves for homework, and then work in the lesson with designed ‘student’ responses to the same problem. The students’ task may be to understand them, make connections between them, evaluate them and so on. The solutions are designed as if written by anonymous students.


Can designed student responses support teachers to interact with students in a productive way?
A report from the Formative Assessment Working Group (2015 ISDDE conference)
Sheila Evans,
University of Nottingham, England
Michal Ayalon, Weizmann Institute of Science, Israel
Abstract
iThis paper introduces the use of pre-designed student responses to unstructured mathematics problems as a possible resource for teachers to develop their capacity of acting contingently in the mathematics classroom in a productive way, whilst teaching. We frame our argument around the notion that contingent moments can be regarded as problem-situations that are, at times, too demanding for the teacher to effectively solve. We provide an unstructured mathematics problem and accompanying designed student responses to illustrate and explain our thinking, considering how this approach has the potential to help teachers flexibly and productively engage with students’ reasoning in-the-moment of instruction. We end the paper with a call for empirical studies to explore these ideas further.
Introduction
iiWhen asked, in a pre-ISDDE conference email (2015), what participants would most like to focus on in the forthcoming formative assessment working group, there was a consensus of opinion. All expressed a wish to explore the design of resources that could help teachers to productively act contingently, in the moment of instruction. This we did. We considered both why behaving contingently can enhance student learning, and why teachers find it challenging. We then began designing resources. Within this process, we added a further dimension. We decided that, rather than designing materials for professional development sessions, we would design resources that provide opportunities for teachers to develop their practice whilst practicing. We converged on one particular design idea, the use of pre-designed ‘student’ responses to unstructured mathematics problems. Our working group therefore focussed not on formative assessment itself, but with the design of resources to help teachers use formative assessment. In this report we describe the work done in the session. We retrospectively use the research literature to support this work and then reflect on the viability of the use of pre-designed ‘student’ responses.
We, the workshop leaders, report here on some of the outcomes of the lively, diverse and enriching discussions. However, before we do this, we formalise these discussions by exploring the literature to ascertain the validity of our endeavours. We do this first by defining what we mean by teachers acting contingently in a productive way. We then reflect on what it takes to do so in the moment of instruction. We focus on the significance of attending to, and building on student reasoning, and within this process, the importance of developing students’ capacity for self-assessment. Next, we outline the challenges of flexibly and productively improvising instruction. We frame our argument around the notion that contingent moments can be regarded as problem-situations that are, at times, too demanding for the teacher to effectively solve. We then explore why professional development sessions, during which teachers consider solutions to these contingent problem-situations, may not alone be sufficient. This is followed by the presentation of our pedagogical tool: several pre-designed ‘student’ responses to a problem, written as if by anonymous student from another class. We consider how these ‘student’ responses may provide opportunities for teachers to develop their practice. We provide an unstructured mathematics problem and accompanying designed ‘student’ responses to explain and justify our thinking. We then reflect on why having teachers develop their practice just whilst practicing may not be an efficient professional development strategy. Teachers may require support away from their own classroom. We end by summarising our conjectures and considering the need for further empirical research.
Teachers acting contingently
iiiIn a lesson a teacher may gather many different forms of evidence about student thinking. For example, evidence comes through observing students and listening to their dialogue as they tackle a problem, by asking questions and listening to their responses, and so on. This evidence may then be used to provide feedback to students in the moment of instruction. Researchers (e.g. Smit and Van Eerde 2011, Visnovska and Cobb 2015) emphasize that this process only succeeds if framed around teachers’ attending to, diagnosing, and building on student’s reasoning rather than attending to their own ideas for next steps. Van de Pol, Volman and Beishuizen (2011) aptly expressed this practice as ‘When teaching contingently teachers are seen to act less directive and not pursue only their own agenda but, instead, respond adaptively to the needs of students’ (p. 54). This practice can take many forms. A teacher may, for example, explore student ideas further, or help them organize their thinking, or draw attention to connections between differing mathematical ideas, or provide counterexamples, or help extend students’ horizons beyond the immediate tasks.
When reviewing a decade of research on student-teacher interaction, Van de Pol, Volman and Beishuizen (2010) proposed that contingent action is the first stage in productive teacher scaffolding of student thinking. A teacher's support is temporary (Wood, Bruner et al. 1976), and as the student makes progress, the support is gradually withdrawn and responsibility for learning is returned to the student. The emphasis is on allowing students to determine for themselves whether to continue with their current strategy or make amendments to it (Smit, Van den Heuval et al. 2013). The teacher’s feedback, for example, may lead students to re-interpret the problem, or/and to adjust their own goals. Within this process, Sadler (1989) identified three essential conditions to enhance learning: students must know what good performance looks like, how current performance relates to good performance, and how to act to close the gap between current and good performance. This observation has led researchers to conclude that, for students to ‘close the gap’, they must possess self-assessment skills (Yorke 2003). Thus it is incumbent on teachers to use contingent moments to enhance these skills.
What does it take to act contingently?
1If students work through routine exercises that foster the memorisation of algorithms, then they may develop procedural fluency, but their reasoning is likely to be minimal. The classroom discourse will inevitably be less open, and opportunities for the teacher to be exposed to, and build on, a wide variety of mathematical ideas is limited. The need to act contingently will still exist. Students will get stuck, ask questions, and so on, but there will be a degree of predictability and uniformity within these contingent moments. On the other hand, if students tackle unstructured problems that can be solved in multiple ways, teachers may encounter varied and novel student approaches. This can provide an abundance of opportunities to support student learning, but it can also be pedagogically challenging. In particular when a student’s reasoning is ‘a work in progress’, includes errors, is not clearly communicated, or employs an unanticipated approach (Evans and Swan 2014). A teacher may experience a tension between maintaining a student’s social status, avoiding insincere praise, ensuring emerging ideas are understandable by peers, and a commitment to advancing all students understanding and performance. The uncertainty of knowing how best to respond to what students say or do, or simply reacting through habit, often results in teachers providing feedback in the form of mathematical information, rather than focusing on students’ own understandings (Piljs and Dekker 2011). A teacher may, for example, tell the student what to do next, make the task simpler, or do part of the task for the student (e.g. Wood 2001). Thus, at least for the current task, the potential for student learning will be constrained.
Many researchers argue that how teachers respond to contingent moments is dependent on their own knowledge (Chick and Stacey 2013). This knowledge includes knowledge of students as students, such as knowing what question is suitable for one student but not for another. It also includes knowledge of the mathematical content, such as how two students’ ideas may be connected (Ball, Thames et al. 2008) and knowledge of the pedagogy of problem solving (Foster, Wake et al. 2014), such as knowing how to facilitate students reflecting on the assumptions they are making whilst tackling a problem. However, these researchers also recognise that although knowing how to interact with students is an essential requisite for productive interaction, it is not an exclusive factor. Knowledge is not simply an inert list of things to be possessed and then transported from one situation to the next (e.g. Mason and Davis 2013). For teachers to flexibly and productively engage with students requires a form of knowing in the moment of instruction. Ball and colleagues (Ball, Thames et al. 2008) termed this kind of knowing as knowledge-in-action. This is quite different from what a teacher may know as represented in an exam or through a discussion in a seminar.
Mason and Davis (2013) went so far as to propose that teacher’s capacity to articulate outside the classroom, the pedagogical strategies they could use in the classroom, is often unrelated to the actual pedagogical strategies they use in the moment of instruction inside the classroom. To support this view they recount a professional development session in which teachers were introduced to a new way of teaching. All participating teachers appeared to understand the new pedagogy and were committed to ‘teaching differently’. Yet when the teachers attempted to enact these new ideas in the classroom, they encountered insurmountable difficulties. Their students did not generate insights similar to the ones they had observed in the ‘model’ lesson. When faced with unforeseen situations, rather than listening to, and engaging in the students reasoning, they all readily resorted to explaining the next steps to solving a problem. The teachers seemed to lack the vital connective tissue between knowing strategies and being able to readily access them when confronted with novel situations, in the moment of instruction. Instead they relied on engrained habits to get through unanticipated, problematic situations. It appeared that talking the talk in one setting was not equivalent to ‘doing’ the talk in another setting.
There could be a number of reasons for these behaviors. The teachers may have indeed lacked knowledge or the commitments to enact the knowledge. Alternatively, the difficulties of integrating ideas and practices learned outside the classroom into practices within the classroom can be considered from the perspective of situated cognition (e.g. Lave and Wenger 1991). Situated theorists regard the physical environment as an integral part of learning, and not just a set of circumstances in which context-independent processes are learnt (e.g. Putnam and Borko 2000). They argue that teacher’s knowledge, like any other form of knowledge, is located in social practice. As such, teacher’s actions and their learning cannot be fully described independently of the specific situation (Lave and Wenger, 1991). From this perspective, the complexities of interactions between teacher, student and mathematics cannot be reduced to individual qualities (e.g. knowledge of a new pedagogical strategy), isolated from one another (Putnam and Borko 2000). Accordingly, teachers’ engagement in new practices within a professional development session will inevitably differ from their interpretations and enactment in the complex setting of the classroom. Seemingly straightforward solutions to improving pedagogy can, in the moment of a problem-situation in the classroom, be unfeasibly difficult to implement.
A Solution: Pre-designed worked-out solutions
ivThis leads us back to our original question: Can we design resources to help teachers develop the practice of acting contingently in a productive way, whilst teaching?
To support our design strategy for the resources we adopted the perspective argued by Stacey (2006): that mathematics teaching is an activity in which teachers apply mathematics and their knowledge of teaching to problem-situations. She claimed:
‘This places the emphasis not on the static knowledge … but on a process account of teaching. … In the case of teaching mathematics, the solver has to bring together expertise in both mathematics and in general pedagogy, and combine these two domains of knowledge together to solve the problem, whether it be to analyse subject matter, to create a plan for a good lesson, or on a minute-by-minute basis to respond to students in a mathematically productive way’. (p. 8–9).
For us, the problems to be solved were in the moment of instruction, when teachers are faced with problematic situations. By tackling these problems, opportunities for teachers to ‘learn in action’ are generated. And like any problem, the perceived degree of difficulty can determine how successfully it is tackled. They should not be so challenging that the teacher resorts to old habits, instead of employing new ones. And they should not be so easy that the problem-situations are routine and provide little potential for the teacher to develop their practice.
Clearly, the degree of difficulty of the contingent problem-situations is influenced by the tasks students work on. As we have already reported, if the task fosters varied approaches in which unforeseen student difficulties arise, the teacher, unsure how to solve their ‘applied mathematical problem’ may find newly learnt pedagogical strategies are not so readily accessible in the moment of instruction. Thus they may resort to familiar, often ‘automatic’ practices, such as telling the student what to do next. In contrast, if students work through exercises practicing the same procedure their approaches, and the struggles they encounter, will be more predictable and similar. The contingent problem-situations, for the teacher to solve, will be correspondingly routine and less cognitively demanding. The range of possible solutions is likely to be familiar and may not require teachers to use their newly learnt pedagogical strategies. In both the routine and open problem-situations teachers will not have the opportunity to ‘learn in-action’ how to productively and flexibly engage with student reasoning. Teachers need to encounter manageable, not too difficult, problem-situations in which newly learnt pedagogical strategies (e.g. feedback questions) are accessible and required in order to productively respond to students in a way that focuses on, and supports their reasoning.
We consider that these designed ‘student’ responses to a problem are a specific form of worked-out examples. The use of worked-out examples is a key pedagogical tool in many instructional settings (Renkl, 2013 #227), including in particular the Mathematics Assessment Project (see Evans and Swan 2014; Swan and Burkhardt 2014). These resources can be found online at: http://map.mathshell.org/lessons.php. Numerous studies demonstrate that exposing students to multiple, carefully selected worked-out examples of varying formats, can be more beneficial to student learning when compared to unaided problem solving (e.g. Cooper and Sweller 1987). In many cases, worked-out examples not only foster student learning, but also reduce the necessary learning time (Salden, Koedinger et al. 2010). Researchers reason that when studying worked-out examples, students are freed from performance demands and can concentrate on gaining understanding (Renkl, Gruber et al. 2003).
Although there is an extensive body of research on the specifics of students’ learning from worked-out examples, there is little (we could find none) on how their use impacts teacher practice. We hypothesize that teachers, like students, will shift their focus away from encouraging students to complete a task, to encouraging them to understand solutions to the task. For example, when a student is stuck, a teacher may focus on their understandings of the completed solution rather than what they have done and what they need to do. We hypothesize also that the implicit focus just on student reasoning may reduce the complexity of improvisation. Moreover, if the designed responses are clear and organised, the in-the-moment demands of interpreting and clarifying often emerging student ideas, is also reduced.
The anonymity of the designed ‘student’ response simplifies contingent problem-situations further. Teachers need not be concerned, when solving a contingent problem-situation, about the social issues of exposing individual students’ incomplete or incorrect thinking. Also, the emotional aspect of students’ publicly describing their own work for others to review is removed. Instead students describe the solution of another, unknown to the class. This can again reduce the demands of improvisation. The teacher can readily ask direct questions that focus on reasoning, such as ‘Do you think the ‘student’ has used an efficient approach?’ without worrying about maintaining the author’s social status or credibility as a mathematician (Evans and Swan 2014). And particularly if the worked-out ‘student’ solutions vary in terms of ‘differentiated quality’ with respect to some specific mathematical features (e.g., quality of argumentation, quality of language, efficiency or generalizability of a solution), students’ criteria for success are made visible for scrutiny (Black & Willam, 1998). Mismatches between teacher and students’ criteria may surface, providing opportunities for the teacher to encourage students to reflect on their success criteria and adapt them to accommodate new values. These experiences may similarly prompt teachers’ to reflect on and review their own criteria for assessing students' authentic solutions. And as previously mentioned, encouraging students’ self-assessment skills is an essential ingredient of effective scaffolding. Thus we argue that encouraging assessment skills when students’ work with anonymous responses may support this practice when students are working with their own solutions.
Because each task is accompanied by a restricted, but known range of ‘student’ approaches, the teacher can plan in a more detailed way, before the lesson, the issues real students may face when working with the designed responses. This preparation will be enriched if teachers also review their own students’ initial attempts to the problem (Evans and Swan 2014). Thus teachers can tackle in planning some of the problem-situations that are normally tackled in the moment of instruction, such as how they could encourage students to make mathematical links between responses, or seek generalizations, and so on. Planning in this way will enable teachers to prepare and explicitly enact, practices that may not readily come to mind when the contingent classroom problem-situations are too demanding. We recognize that planned for and then enacted instructions do not equate to contingent instruction. However there exist greater similarities of ‘knowing in action’ between the two student classroom settings (students working with authentic solutions and students working with designed student responses) than between the professional development and the classroom setting. As Hodgen (2011) suggests it is the recognition of the similarities and differences between patterns of thought and action that enables transfer of knowledge between settings. Thus we speculate that experiences within the less demanding setting of students working with designed ‘student’ responses may facilitate the transfer of developed practices to the more demanding, contingent problem-situations occurring when students solve problems for themselves. There may still be unanticipated contingent moments when students work with designed ‘student’ responses, but because the complexities of improvisation are reduced, we speculate that many in the moment problem-situations will be effectively solved by the teacher, thereby providing further opportunity for the teacher to develop their practice whilst practicing.
We are not suggesting it is better for students to work with these responses than to work with their own solutions to a problem. Both provide opportunities for student learning. However the experience of using designed ‘student’ responses may place teachers in a better position to generalize their ‘knowing-in-action’ to the more demanding setting of students’ solving unstructured problems for themselves.
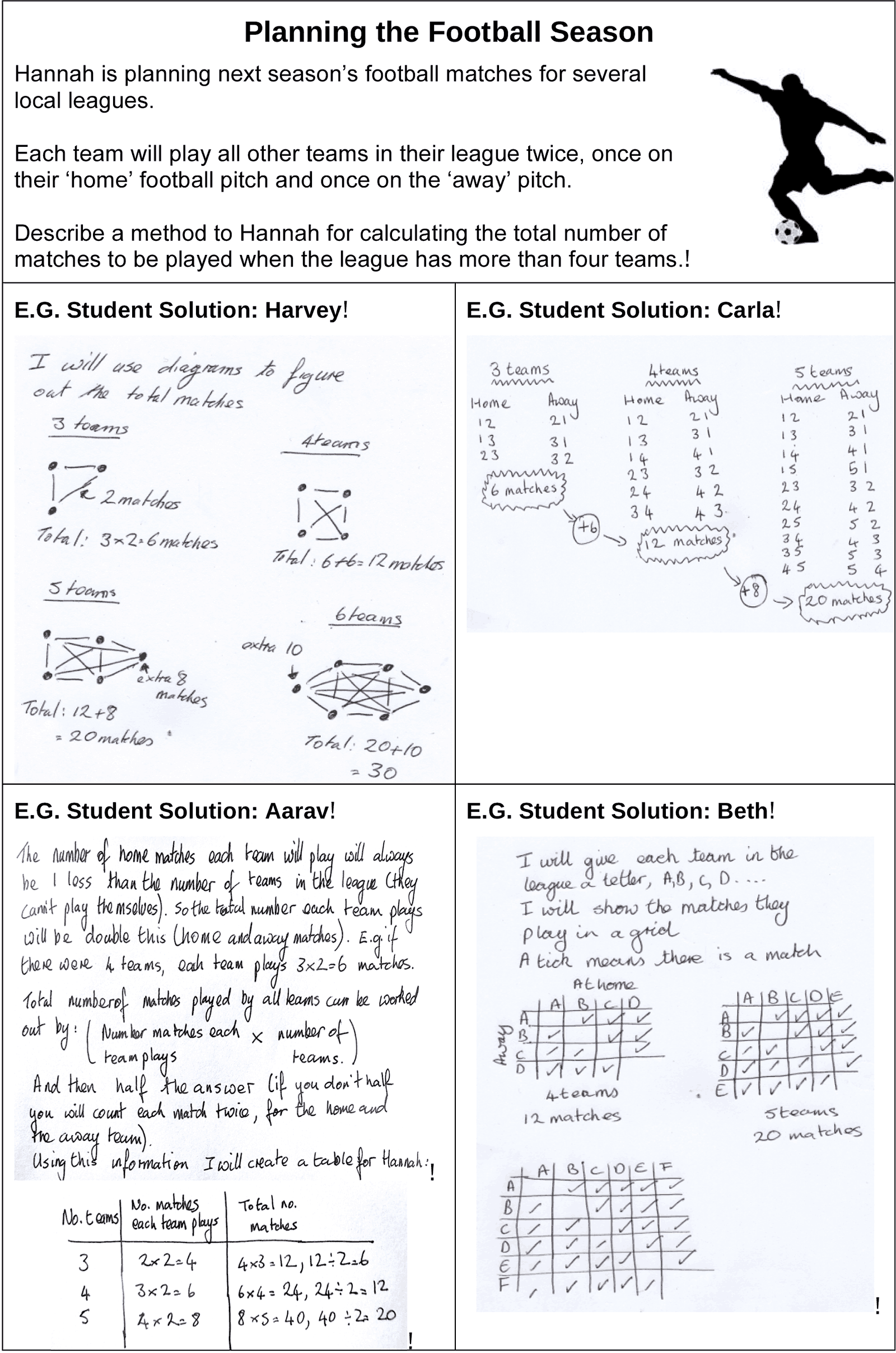
Design intentions: Planning a football season
1We will now explain in detail our thinking behind the example provided in Figure 1. The solution goal of the problem ‘Planning a football season’ is reasonably clear, but because the route to this goal is not specified, there are many different solution strategies. When implementing such a problem, teachers need to simultaneously be attentive to the ways students understand and approach the problem, the features students attend to, and the language they use in communicating them. Students' ideas can be ambiguous, not clearly articulated or not anticipated. This can cause teachers to encounter many demanding problem-situations in the moment of teaching.
In concordance with the design of the lessons within the Mathematics Assessment Project (see Swan and Burkhardt 2014), we recommend that students attempt the problem first, for homework. This provides the teacher with the opportunity to review student work, and so be better informed about students’ understandings. In the lesson, students are then presented with two or more designed ‘student’ responses. Based on our own experiences as teachers and research designers, we expect that when real students tackle the problem for themselves, many will use a similar (although possibly less structured) strategy to Carla’s numeric response. Its deliberate inclusion may facilitate students’ understanding of the other responses (Ainsworth 2006). The other three solutions were designed for a number of reasons, including: for students to appreciate how a situation can be represented diagrammatically in various ways, to provide opportunities for students to recognize how relationships between variables can be used in contrasting ways, to support students making connections between solutions, and to encourage students to employ different methods in the pursuit of generalizations.
Contingent problematic situations for the teacher could occur when students struggle to understand a solution, or possess an ‘illusion of understanding’ (Chi, Bassok et al. 1989). For example, students may be able to interpret Beth’s solution, but not understand how it was constructed, or they may be able to correctly add a further row to Aarav’s numeric table but not appreciate how the table relates to the context. Research shows that prompting students to explain the solutions can facilitate their understanding (Renkl 1999). Teachers could prepare for these situations by developing questions, before the lessons that focus on student reasoning. For example, questions to promote reasoning and mitigate the ‘illusion of understanding’ could be: “Why does Aarav multiply 8 by 5 in the third row of the table?” or “What do the dots represent in Harvey’s solution?”
The teacher could encounter other challenging situations if the students are asked to make connections across solutions. Again teachers can prepare ways of supporting students through questioning and prompts. This could include directing students’ attention to the structural features that remain constant across problems (e.g. Berthold and Renkl 2009). Examples include ”How are the dashes in Harvey’s solution represented in Beth’s solution?”, “What makes Aarav’s approach strategically different from Beth’s solution?”, “How is each column/row of Beth’s solution represented in Aarav’s solution?”, “What are the similarities between Carla’s and Harvey’s solutions?” The teacher may also prepare questions to encourage students to move towards generalizations, such as “Can you think of a way to extend a solution so that Hannah could use the method for any number of teams?” Furthermore, the teacher may ask students to compare and evaluate the methods. By focusing their instruction on a particular criteria, for example, the potential for generality, the efficiency, or the clarity of solutions will help students (and also the teacher) appreciate what good performance looks like.
Through careful preparation the teacher may encounter in the lesson manageable contingent problem-situations that can be solved by focusing on student reasoning. These experiences may in turn help the teacher develop their practices whilst practicing. Moreover, the questions the teacher prepares can be asked not just within a contingent problem situation, but whenever there is an opportunity for student learning. In so doing it will help foster practices that the teacher may then transfer to the more demanding situation of students solving unstructured problems for themselves.
Will learning new practices by practicing be sufficient?
vAlthough we have argued for teacher learning whilst teaching, in agreement with other researchers (e.g. Mason and Davis 2013), we recognize that teachers learning just from experiences in the classroom may be an inefficient approach to professional development. Furthermore, as Putman and Borko (2000), noted, because many teachers’ responses to problematic-situations are engrained and automatic, they can be resistant to reflection or change. Engaging in learning experiences away from the classroom may be a necessary way of introducing and reflecting on new ways of acting contingently and at the same time help teachers break with old habits.
We do not regard suggesting the use of professional development sessions conflicts with our perspective on situated learning. While we recognise the difficulty of transferring knowledge between divergent physical settings, we take the view argued by Putnam & Borko (2000, p. 13): learnt principles are ‘intertwined collections of more specific patterns [of thought and action] that hold across a variety of situations’.
Thus we propose that while the differences between professional development sessions and the classroom can inhibit transfer, the introduction of designed ‘student’ responses may help mitigate the differences. We argue that the threads of knowledge weaving through the mathematics classroom and the professional development classroom may engender transfer of ‘knowing’ and ‘knowing in action’ between the two settings.
Discussion
viWe recognize that teachers improvising in a productive manner can support student learning, but this can be challenging to implement, particularly when students are solving problems in unanticipated ways, are stuck, or their ideas are emerging. The contingent problem-situations may be too difficult for teachers to productively solve in the moment of instruction. In this paper we proposed a strategic pedagogical instrument, called designed ‘student’ responses, as the focus of an instructional method for improving teacher’s capacity to act contingently. The design of this tool is framed around the perspective that the potential for teacher learning in the classroom is dependent on opportunities to ‘learn in action’. These opportunities can arise when teachers practice newly learnt pedagogies, including effectively solving contingent problem-situations.
We hypothesize that detailed planning before a lesson, in a way not possible when students are solving a problem for themselves, together with the knowledge gained in a professional development setting may be mapped more readily onto the planned for instruction and contingent moments when students are working with designed ‘student’ responses. Factors such as the restricted number of solutions, their anonymity and the focus on understanding solutions rather than on performance, all contribute to reducing the complexity of improvisation. The contingent problem-situations are more manageable and provide teachers with feasible opportunities to productively solve them. Thus teachers may develop their practice of productively solving contingent problem-situations whilst practicing. These developed practices can then be transferred to the more challenging, but clearly analogous, environment of students working with their own solutions. Ultimately, this may render the more difficult in the moment problem-situations solvable by the teacher.
We view our discussions, both in the workshop and in this paper, as a preliminary overview of the supporting research into how the instrument could be used to help teachers flexibly and productively engage with students’ reasoning in the moment of instruction. There is a need for empirical studies to explore these ideas further.
Acknowledgements
viiWe would like to acknowledge the contributions made to this paper by participants of the 2014 and 2015 ISDDE Conference Formative Assessment Working Groups:
Riana Adams, Harold Asturias, Anna Baker, Mac Cannady, Elizabeth Coyner, Lizzie Kimber, Judith Kysh, Will Morony, Minoru Ohtani, Daniel Pead, Monique Pijls, Kevin Reins, Tom Sallee, Max Stephens, Malcolm Swan, Betsy Taleporos, Karen Wootton, and Dan Zalles.
References
viiiEvans, S., Swan, M. (2014) Developing Students’ Strategies for Problem Solving. Educational Designer, 2(7). Retrieved from: http://www.educationaldesigner.org/ed/volume2/issue7/article25/
Swan, M., Burkhardt, H. (2014) Lesson Design for Formative Assessment. Educational Designer, 2(7). Retrieved from: http://www.educationaldesigner.org/ed/volume2/issue7/article24/
About the Authors
ixSheila Evans is a member of the Mathematics Assessment Project team in the Centre for Research in Mathematical Education at the University of Nottingham. For the last four years she has worked designing, observing, teaching, revising and providing professional development for the MAP Formative Assessment Lessons. She is currently working on a doctorate using teaching resources that have been shaped by this project. Before that, she taught for fifteen years in secondary schools in the UK and Africa, and wrote a textbook Access to Maths aimed at students without traditional qualifications who wished to study at University .
Michal Ayalon is a Senior Intern in the Science Teaching Department, the Weizmann Institute of Science, Israel. She holds a Ph.D. in math education from WIS, and served as a postdoctoral fellow at the Department of Education, University of Oxford, England. Her interests lie in learning on, and promoting of, teaching practices in the mathematics classroom, in particular argumentation and formative assessment. Michal is the director of the Mesimatica project for developing andsupporting the use of formative assessment tasks, covering the advanced track (5 units) of the Israeli 10th grade mathematics curriculum.